|
Is the Quality Controlled?
Sample from the First Batch
A baker aims to make 400-gram loaves. To find whether he is
producing overweight or underweight loaves, he weighs a sample of
10 loaves from his first batch. His results (in grams) were:
| 415 |
394 |
408 |
422 |
428 |
| 397 |
425 |
406 |
414 |
421 |
The weights of the loaves were all different. To find the MEAN
(average) weight of the loaves, we use:
| MEAN weight = |
| (Total weight of all
loaves) |
|
- What is the total weight of these 10 loaves?
- Find the mean weight of these 10 loaves.
The loaf weighing 415 grams contained an extra 15 grams of
bread. The loaf weighing 394 grams was 6 grams short. In these
two loaves, the total weight of extra bread was 15 - 6 = 9 grams,
i.e. the total overweight of these two loaves was 9 grams.
The weights of these loaves are recorded in Table 4 on page
R1.
- Complete the table by subtracting 400 from the
weight of each loaf. The first two examples have been
done for you. The second one is negative. What does this
mean?
- What is the total overweight of the 10 loaves?
- Use your answer to d to find the mean overweight
of the 10 loaves.
- Write down another method of working out the mean
overweight. (Hint: look at your answer to b.)
Can you think of a way of measuring the variation in
weight of these 10 loaves?
One way is to subtract the smallest from the largest. The
result is called the RANGE.
- What is the weight of the heaviest loaf.
- What is the weight of the lightest loaf.
- What is the range (in weight) of these loaves?
- How much overweight is the heaviest loaf.
- How much overweight is the lightest loaf (it is
negative)?
- What is the range in amounts overweight?
- How does your answer to 1 compare with that to i?
A Full Day's Work
You will need a sheet of graph paper.
The baker weighs a sample of 10 loaves every hour. The weights
from the first sample have already been given in the previous
Section. Each hour he works out the mean weight of his sample. Table 2 gives the eight sample means obtained
during the day.
Table 2 - Mean weights of
loaves.
The baker was aiming to produce 400-gram loaves. In sample 3,
the mean weight was only 396 grams, so the mean overweight was
396g - 400g = - 4 grams.
- Is the mean overweight of any other sample
negative?
- Fill in the mean overweights of the eight samples
in Table 5 on page R1.
The mean overweight of each sample can be plotted on a QUALITY
CONTROL CHART like the one below. Notice that the zero line is
where the mean weight of the sample is exactly 400 grams.
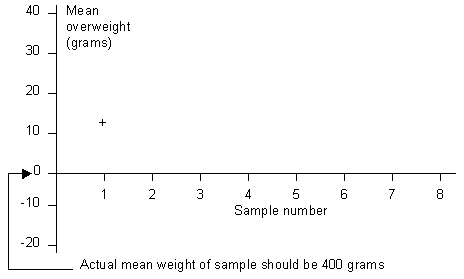
Figure 1 - Quality control chart mean overweights
of eight samples of loaves.
- Draw the axes for a quality control chart, like
the ones above, on your piece of graph paper.
- Plot the mean overweights of each sample that you
found in b.
The first point has already been plotted on the chart above.
The baker knows the usual pattern of points on his chart. He
can quickly see when something goes wrong.
What do you think he looks for?
- Look at the last three samples. Are the loaves
generally too heavy, too light or about right?
- For part of the day, the mean weight of
successive samples decreased. Which samples were these?
- After which sample do you think that the baker
made some adjustments? What effect did they have?
*Are There Enough Matches In a Box?
A firm sells matches in boxes marked 'Average contents 40
matches'. A sample of 10 boxes was taken from the production line
every hour. The contents of each box were counted. The mean
number of matches per box was calculated. The results are given
in Table 3.
Table 3 - Mean number of
matches per box.
The zero line on the quality control chart for these figures
represents 40 matches. The vertical axis goes from -3 to +3 and
is labelled 'Mean number of matches over 40'.
- Draw the quality control chart for the eight
samples.
Use the chart to answer the following questions:
- In which sample do you think that too many
matches might have been put into the boxes?
- Comment on any general 'trend'.
- What sort of action, if any, should the
production manager take to correct any possible faults?
- Each box is marked 'Average contents 40 matches'.
Is this a fair claim? Give your reasons.
- Look back to the quality control chart you drew
in Section C2 for the loaves. Compare it with the one you
have drawn for the matches. What differences do you
notice?
|